
Understanding D.C. Motor Characteristics

Home Intro How They Work CHARACTERISTICS Examples

This page has been requested times.



This site deals with Direct Current permanent magnet motors operated at a constant voltage. Motor characteristics vary considerably from type to type, and their performance characteristics can be altered by the way electrical power is supplied. can be quite different than those covered here.
 is typically measured in
radians/second {rad/s},
revolutions/second {rps},
or revolutions/minute {rpm}.
is typically measured in
radians/second {rad/s},
revolutions/second {rps},
or revolutions/minute {rpm}.
|
1 revolution = 360° 1 revolution = (2*p) radians 1 radian = (180/p)° 1° = (p/180) radians |
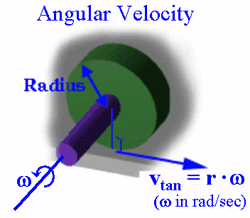 From the angular velocity,
From the angular velocity, 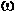 , we can find the tangential velocity of a point anywhere on the
rotating body through the equation tangential velocity, v = r*
, we can find the tangential velocity of a point anywhere on the
rotating body through the equation tangential velocity, v = r*
 , where r is the distance from the axis of rotation. This relation can be used to compute the
steady state (constant speed - no acceleration) speed of a vehicle if the radius and angular velocity of a
wheel is known, or the linear speed of a rope as it is wound up by a winch.
, where r is the distance from the axis of rotation. This relation can be used to compute the
steady state (constant speed - no acceleration) speed of a vehicle if the radius and angular velocity of a
wheel is known, or the linear speed of a rope as it is wound up by a winch.
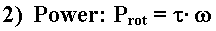
| SI | English |
|
Watts {W} newton-meters per second {N·m/s} 1 W = 1 N·m/s 1 W = 0.738 ft·lb/s 1 W = 1.341E-03 hp |
foot-pounds per second {ft·lb/s} horsepower {hp} 1 ft·lb/s = 1.818E-03 hp 1 ft·lb/s = 1.356 W |
![[Characteristic Torque/Speed Curve for a D.C. Motor]](colorTS1.jpg)
![[Ts]](Ts.gif) , represents the point on the
graph at which the torque is a maximum, but the shaft is not rotating.
, represents the point on the
graph at which the torque is a maximum, but the shaft is not rotating.
![[Wn]](Wn.gif) , is the maximum output
speed of the motor (when no torque is applied to the output shaft).
, is the maximum output
speed of the motor (when no torque is applied to the output shaft).
![[3) T=Ts-W*Ts/Wn; 4) W=(Ts-T)*Wn/Ts]](eqn3-4.gif)
|
The linear model of a D.C. motor torque/speed curve is a very good approximation. The torque/speed curves
shown below are actual curves for the green maxon motor (pictured at right) used by students in 2.007.
One is a plot of empirical data, and the other was plotted mechanically using a device developed at MIT.
Note that the characteristic torque/speed curve for this motor is quite linear. This is generally true as long as the curve represents the direct output of the motor, or a simple gear reduced output. If the specifications are given as two points, it is safe to assume a linear curve. |
![[green maxon motor used in 2.007]](gmaxonthumb.gif) |
![[empirical torque/speed curve]](gmaxonts.gif)
![[mechanically drawn torque/speed curve]](finalpeterzi.gif)
|
|
 = ½
= ½
 , and
, and  = ½
= ½
 .
.![[power represented as area under torque/speed curve]](colorTS3.jpg)
![[power represented as area under torque/speed curve]](colorTS2.jpg)
![[power represented as area under torque/speed curve]](colorTS4.jpg)
![[5) P(W)=-(Ts/Wn)*W^2+Ts*W]; 6) P(T)=-(Wn.Ts)*T^2+Wn*T]](eqn5-6.gif)
![[T]](T.gif) = ½
= ½ , and
, and
![[W]](W.gif) = ½
= ½ repectively.
repectively.
![[D.C. motor power vs. torque curve]](torquepowerspeed.jpg)